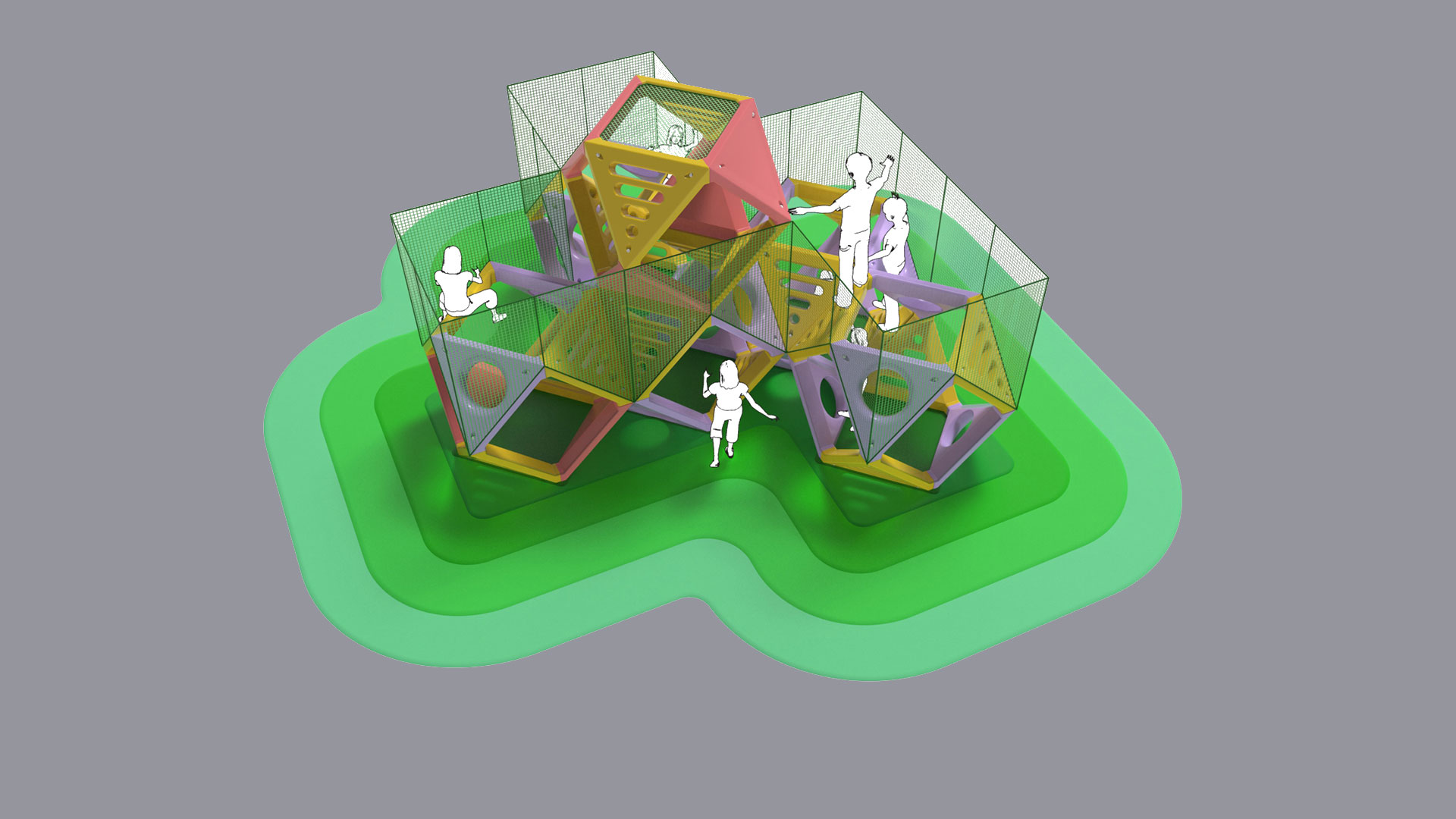
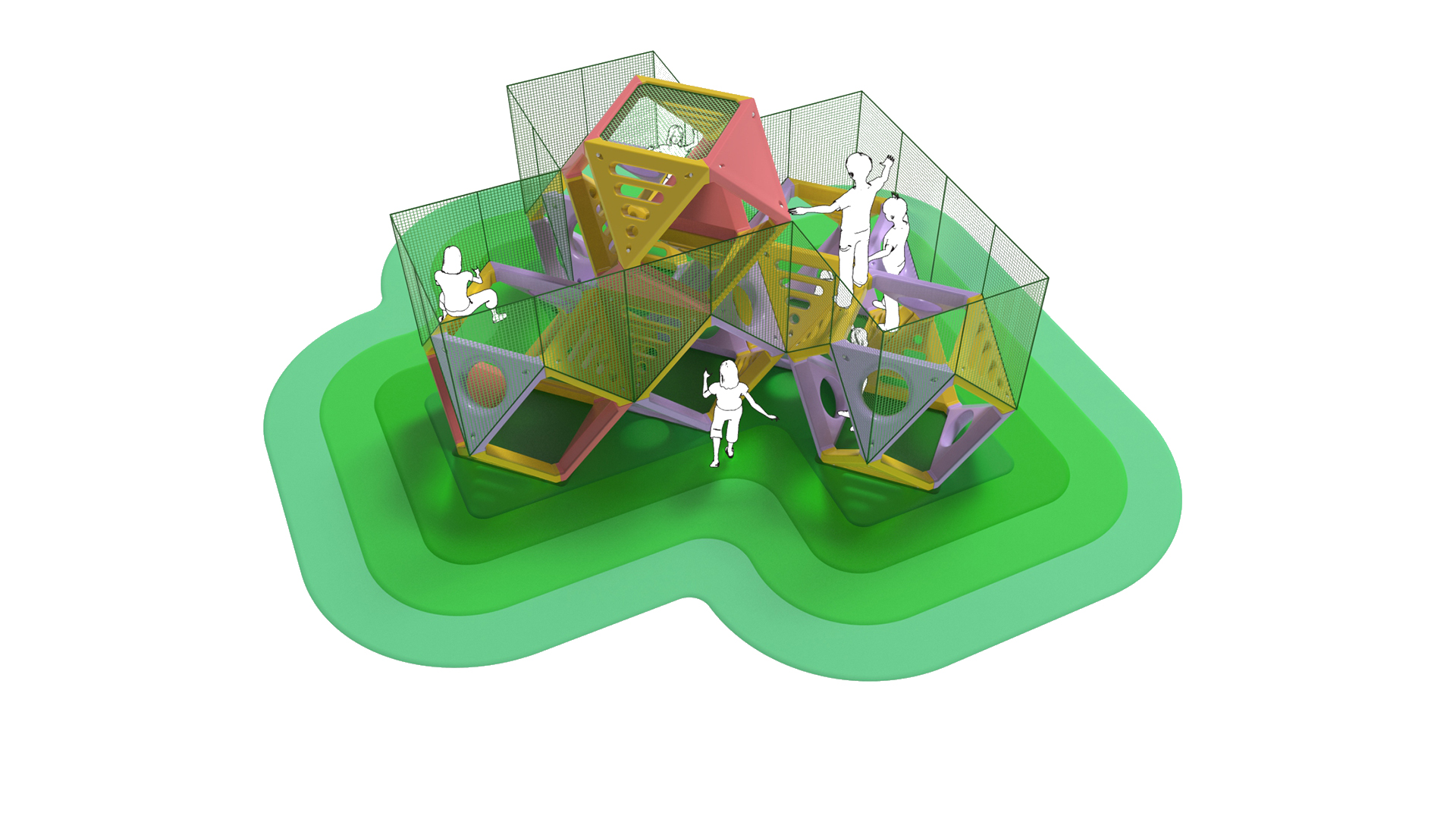
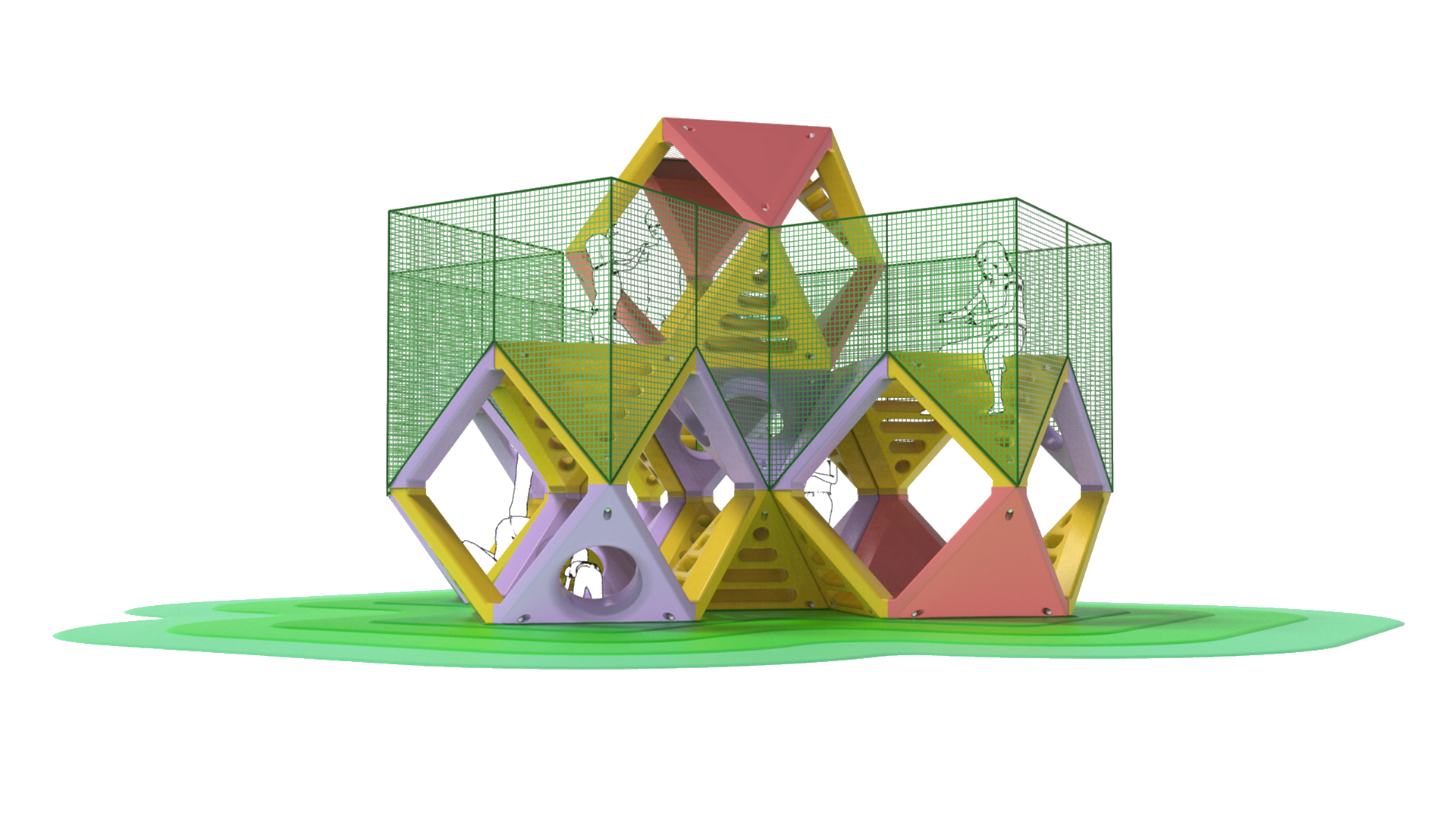
We owe to Archimedes, the ancient Greek mathematician and scientist, the original shape that serves as the basis for the design of this new children’s game. It is said that it was in the 3rd century BC that Archimedes discovered a particular series of 13 polyhedra, known today as “Archimedean solids” or, also, semi-regular polyhedra. These are convex polyhedra resulting from the combination of regular polygons of two or more types. One of their most relevant characteristics is that all their vertices are circumscribed in a sphere. From these 13 archimedean solids we have chosen the cuboctahedron as the main component of the new children’s play design. The cuboctahedron is the result of truncating a cube to the midpoint of its edges, therefore obtaining 14 faces: the 6 of the cube, which remain square, and 8 new ones in the form of equilateral triangles. With this semi-regular polyhedron we have created Polyhedric Land.
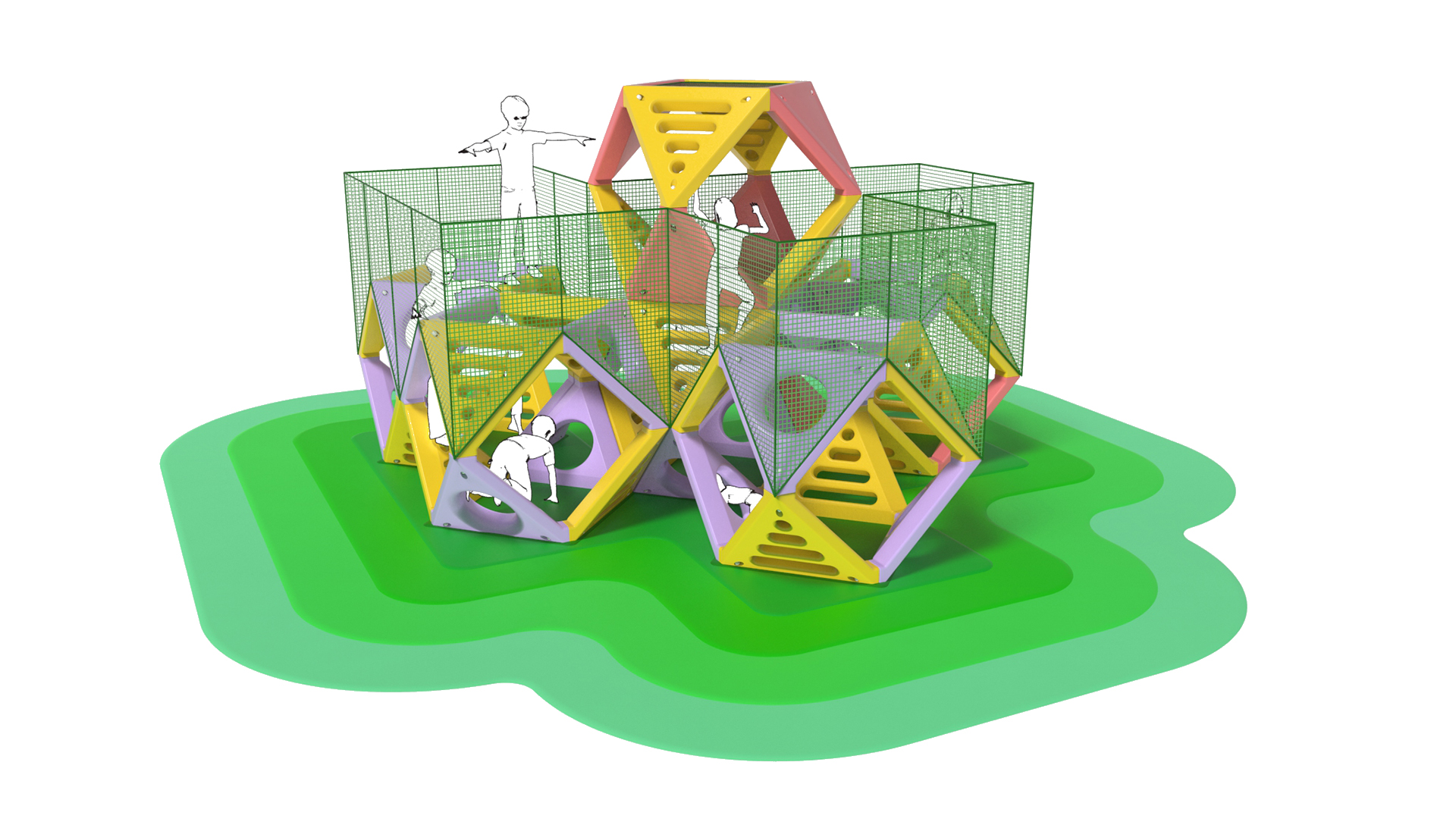
Polyhedric Land is a self-supporting and scalable modular game for children, based on the repetition of a 1.5 metre high cuboctahedron. By configuring it in line or by stacking it, we achieve a three-dimensional labyrinthine space. In addition, each of the sides of the cuboctahedron is hollowed out in the form of grooves or circular holes, which, together with the smooth sides, adds new potential to the game. Depending on the type of polyhedron grouping, this modular play system provides for elevated perimeter safety nets.
As a result of our design, Polyhedric Land, as we have presented it, is a territory where children can climb, clamber, explore inside, hide and perform many other feats, wherever their imaginations and their desire for fun lead them. In this way, without realising it, they develop their psychomotor and collaborative skills. Although its design is based on the mathematics and geometry of an ancient genius, the little ones only need their desire for adventure to enjoy Polyhedric Land.